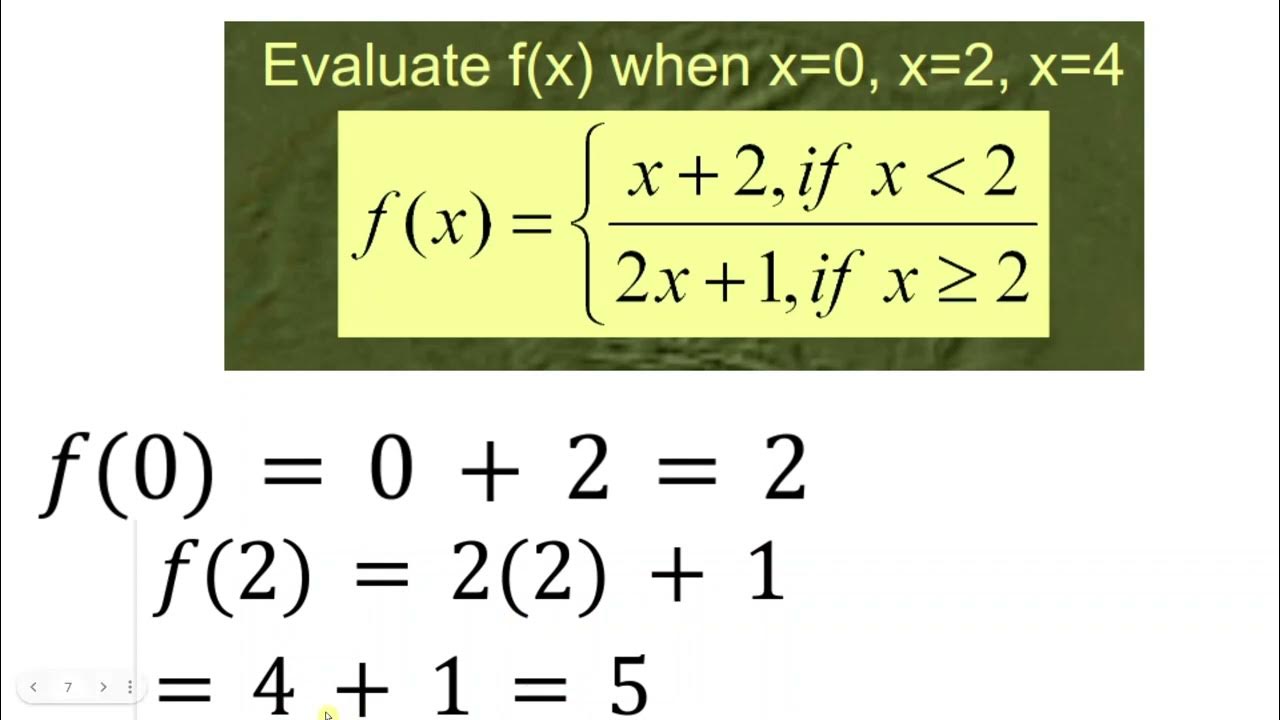Understanding The Intricacies Of Symbolab Piecewise Function Inverse. 5

Mathematics is often perceived as a complex labyrinth, particularly when it comes to functions and inverses. Among the various topics that students encounter, piecewise functions stand out as a unique and intriguing area of study. These functions, defined by multiple sub-functions over different intervals, can sometimes seem daunting. However, with tools like Symbolab, tackling the intricacies of piecewise functions and their inverses becomes significantly more manageable. The focus of this article is to delve deeper into the concept of the Symbolab piecewise function inverse. 5, providing clarity and understanding to those eager to master this mathematical concept.

The Symbolab platform is an excellent resource that enables students and learners to visualize and compute mathematical functions, including piecewise functions and their inverses. The piecewise function inverse is particularly interesting because it allows us to understand how to reverse the operations of a function defined in segments. By using Symbolab, one can efficiently determine the inverse of a piecewise function, gaining insights into its behavior and properties. In this article, we will explore the definition, characteristics, and applications of piecewise functions, along with detailed instructions on using Symbolab for finding their inverses.

As we embark on this mathematical journey, we will address common questions such as: What exactly is a piecewise function? How do we determine the inverse of such a function using Symbolab? What are the practical applications of piecewise function inverses in real-world scenarios? By the end of this discussion, readers will not only grasp the concept of Symbolab piecewise function inverse. 5 but also become proficient in applying these principles to solve complex problems.

What is a Piecewise Function?

Piecewise functions are defined by different expressions based on the input value. This means that the rule for the function changes depending on the range of the input. Here are some key characteristics of piecewise functions:

• They can be linear, quadratic, or even more complex functions depending on the interval.
• Each sub-function can have its own domain, allowing for flexibility in modeling real-world scenarios.
• They are useful in various fields, including economics, engineering, and computer science.

How to Create a Piecewise Function?

Creating a piecewise function involves defining different expressions for various intervals of the input variable. For example:

Let f(x) be a piecewise function defined as:

f(x) =
{
2x + 3,   if x < 0
x^2 - 1,   if 0 ≤ x ≤ 2
5 - x,   if x > 2
}

This function uses three different expressions based on the value of x. Understanding how to construct such functions is crucial for analyzing their behavior.

What is the Inverse of a Piecewise Function?

The inverse of a function is another function that "reverses" the action of the original function. For a piecewise function, finding the inverse involves determining the segments that correspond to each part of the original function. This requires solving for x in terms of y for each piece. Let's consider the previous example:

To find the inverse, we break it down:

• For y = 2x + 3, solve for x.
• For y = x^2 - 1, solve for x in the valid range.
• For y = 5 - x, solve for x.

How Does Symbolab Assist with Piecewise Function Inverses?

Symbolab is an online mathematics tool that simplifies the process of finding inverses of piecewise functions. Here's how to use it:

1. Input the piecewise function into the Symbolab interface.
2. Select the option to find the inverse.
3. Review the output, which will include the inverse function and any necessary domain restrictions.

What are the Steps to Find Symbolab Piecewise Function Inverse. 5?

To find the Symbolab piecewise function inverse. 5, follow these steps:

1. Identify the piecewise function you want to invert.
2. Enter the function into Symbolab’s calculator.
3. Analyze the results provided.

Why are Piecewise Function Inverses Important?

Understanding the inverses of piecewise functions is vital for various reasons:

• They allow us to solve equations where the original function is not easily invertible.
• They aid in understanding real-world applications, such as optimizing processes in engineering.
• They enhance problem-solving skills by encouraging logical thinking.

Real-World Applications of Piecewise Function Inverses

Piecewise function inverses have numerous applications in real-world scenarios, such as:

• Modeling shipping costs that change based on weight brackets.
• Analyzing pricing models that differ based on quantity purchased.
• Understanding tax brackets where different income levels are taxed at different rates.

Can Symbolab Handle Complex Piecewise Functions?

Symbolab is equipped to handle a wide range of piecewise functions, from simple linear functions to more complex polynomial and trigonometric expressions. The platform provides detailed step-by-step solutions, making it easier for users to comprehend the underlying principles and processes involved.

Conclusion: Mastering the Symbolab Piecewise Function Inverse. 5

In conclusion, mastering the Symbolab piecewise function inverse. 5 is an essential skill for anyone studying mathematics. By understanding how to define piecewise functions, find their inverses, and utilize tools like Symbolab, students can enhance their mathematical proficiency and apply these concepts in various practical situations. With practice and the right resources, anyone can conquer the complexities of piecewise functions and their inverses.