Understanding The Commutative Property Through Engaging Examples

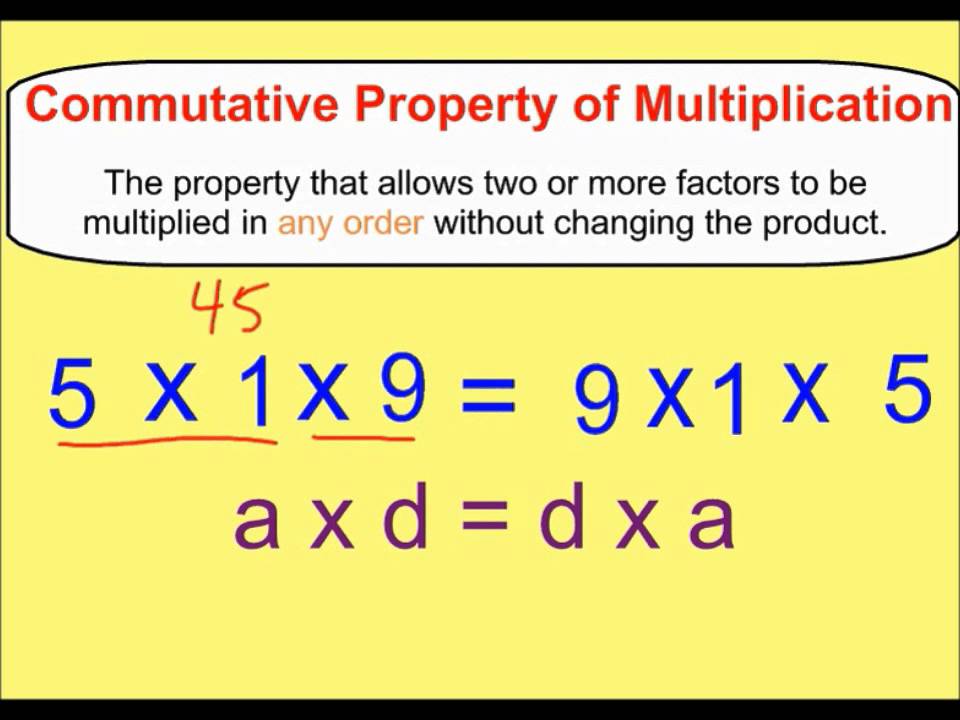
The commutative property is a fundamental concept in mathematics that lays the groundwork for understanding operations such as addition and multiplication. By definition, the commutative property states that the order in which two numbers are combined does not affect the final result. This principle can be observed in everyday life and serves as a crucial building block for more complex mathematical concepts. Understanding examples of the commutative property can help clarify its significance and application in various scenarios.

The beauty of the commutative property lies in its simplicity and universality. Whether you are adding two numbers or multiplying them, the outcome remains unchanged regardless of the order of the numbers involved. This property is not only limited to arithmetic but extends to algebra and higher-level mathematics as well. Grasping these examples of the commutative property will enhance your mathematical skills and provide a deeper appreciation for its utility.

As we delve deeper into the world of mathematics, we will explore various relatable examples of the commutative property. From basic arithmetic operations to more advanced applications, this article will serve as a comprehensive guide to understanding how this property operates in different contexts. Let’s embark on a journey to uncover the fascinating world of the commutative property!

What is the Commutative Property?

The commutative property primarily applies to two operations: addition and multiplication. When we say that addition is commutative, we mean that for any two numbers, say a and b, the equation a + b will yield the same result as b + a. Similarly, for multiplication, a × b will equal b × a. This property holds true for all real numbers, making it a crucial concept in mathematics.

Examples of the Commutative Property in Addition

To illustrate the commutative property in addition, let’s consider some examples:

• Example 1: 3 + 5 = 5 + 3 = 8
• Example 2: 12 + 7 = 7 + 12 = 19
• Example 3: 100 + 25 = 25 + 100 = 125

As seen in these examples, regardless of the order in which the numbers are added, the sum remains the same.

How Does the Commutative Property Apply in Real Life?

In real life, the commutative property can be observed in various situations. For instance, if you have five apples and three oranges, you can say you have eight pieces of fruit, whether you count the apples first or the oranges. This property simplifies calculations and enhances our understanding of quantities.

What Are Some Examples of the Commutative Property in Multiplication?

Similar to addition, multiplication also follows the commutative property. Here are a few examples:

• Example 1: 4 × 6 = 6 × 4 = 24
• Example 2: 9 × 3 = 3 × 9 = 27
• Example 3: 15 × 2 = 2 × 15 = 30

This reinforces the idea that the product remains unchanged, regardless of the order of the factors.

Can the Commutative Property Be Found in Other Mathematical Operations?

While the commutative property is primarily associated with addition and multiplication, it’s essential to note that it does not hold true for all mathematical operations. For example, subtraction and division are not commutative. This means that:

• For subtraction: 5 - 3 ≠ 3 - 5
• For division: 10 ÷ 2 ≠ 2 ÷ 10

This distinction highlights the unique nature of the commutative property and its specific application in mathematics.

How Does the Commutative Property Enhance Mathematical Learning?

Understanding the commutative property is vital for students as it lays the foundation for more complex mathematical concepts. By recognizing that the order of numbers does not matter in addition and multiplication, students can simplify problems and build confidence in their mathematical abilities. This understanding aids in mental math and can lead to a more intuitive grasp of mathematics.

What Are Some Fun Activities to Teach the Commutative Property?

Engaging students in fun activities can enhance their understanding of the commutative property. Here are some interactive ways to teach this concept:

• Number Games: Use dice to roll two numbers and have students practice adding or multiplying them in different orders.
• Real-Life Scenarios: Create word problems involving the commutative property, such as sharing snacks or arranging objects.
• Flashcards: Make flashcards with different numbers and challenge students to find pairs that satisfy the commutative property.

Conclusion: Why is the Commutative Property Important?

The commutative property is a fundamental concept in mathematics that significantly impacts how we approach problem-solving. By understanding examples of the commutative property, students and learners can simplify their calculations and develop a more intuitive understanding of mathematics. This property serves as a crucial building block for more advanced mathematical concepts, making it an essential topic for students of all ages.

In summary, the commutative property of addition and multiplication offers an essential insight into the world of mathematics. Its applicability in real-life scenarios further emphasizes its importance. By exploring engaging examples of the commutative property, learners can deepen their understanding and appreciation for this foundational mathematical principle.